Paying Off Your Loans: Loan Amortization
Long-term loans are important financial tools that can be used to purchase costly capital investments that would otherwise be difficult to obtain by a cash payment. These long-term loans are used in agriculture when purchasing farm equipment, land, or housing. Generally speaking, a long-term loan is a loan that is paid off over an extended period of time of more than a year.
A long-term loan has two components: principal and interest. The principal is the original amount of money that you borrow. If you take out a $100,000 loan, that $100,000 is the principal amount. Interest accrues based on the interest rate, set by the terms of the loan, the amount of principal remaining, and the length of the loan. These factors can significantly impact the total amount of payments made over the lifetime of the loan. Therefore, when securing a long-term loan, it is important to consider not just the payments per period, but also the overall total payments across the life of the loan. This will ensure that a borrower secures the appropriate loan. The purpose of this publication is to provide a guide for those considering a long-term loan.
What Is Loan Amortization?
Loan amortization is the established schedule for paying off a loan over time through regular payments. The loan amortization schedule tells you the beginning period loan balance, amount of the regular payment, amount allocated to the interest, amount allocated to the principal, and remaining loan balance. The principal amount, the stated interest rate, and the length of the loan will all impact the total payments made over the lifetime of the loan.
The most common loan payment schedule is the fixed payment schedule, where each payment made is the same across the amortization schedule. This will be the number that your lender shows you when you take out a loan. The fixed payment amount depends on the loan amount, interest rate, length of loan, and number of payment periods per year. The fixed payment can be calculated by the following equation:
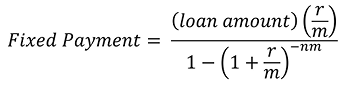
r = interest rate
n = length of loan in years
m = pay periods per year
The interest rate (i) is expressed as a decimal, so an interest rate of 3% would be 0.03. The length of the loan (n) is the years of the loan. The pay periods per year (m) is dependent on how many payments are made each year. For example, if payments are made monthly, then m=12. But if payments are made annually, then m=1.
For each payment made, a portion of the payment goes towards paying interest first and the rest towards paying the principal. In most long-term loans, the interest rate will be based on a fixed interest rate. It means that the interest rate charged for the principal balance does not change over time. However, there can be adjustable interest rates or floating interest rates. Under the adjustable interest rate, the loan rate may fluctuate from time to time. Due to the risk associated with the adjustable interest rate, in general, adjustable interest rate will be lower than the fixed rate. But again, depending on the economic conditions, the interest rate can go up or down, affecting your payment amount per period. This publication focuses on a fixed payment rate; therefore, we only examine a fixed interest rate.
The interest payment depends on the beginning principal balance for the period. This means that the amount of the fixed payment that goes towards interest changes each pay period. At the beginning of the loan a greater portion of the fixed payment will go towards paying interest than at the end of the loan. The interest payment per pay period can be calculated in this way:
Interest Payment Per Period = Beginning of period principal balance * ( r/m )
The difference between the fixed payment amount and the interest payment amount will be used to pay off the principal. The principal payment for each period is calculated in this way:
Principal Payment Per Period = Fixed Payment - Interest Payment
As with interest, the amount of the fixed payment that goes towards the principal will change each pay period. But, in this case, the amount of the fixed payment that goes towards paying the principal increases as more payments are made.
Loan Amortization Example 1
Let’s look at an example of a loan amortization schedule. A producer is looking at taking out a $30,000 loan. The loan is for 5 years to be paid annually with a 5% interest rate. Therefore, the amortization schedule will look like Table 1.
The beginning of period balance is how much of the principal is left to pay for a given period. So, in year 1 period 1, it would be the amount of the loan. In the following periods, it is equal to the ending period balance of the previous period.
The fixed payment amount is how much each payment would be and would be calculated in this way:
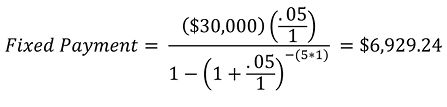
Since this loan is for 5 years paid annually, there would be 5 total payments. Interest and principal payments are calculated for each pay period from this fixed payment amount.
As shown before, the interest paid for each period is dependent on the beginning period balance, the interest rate, and the number of payments per year. For example, in year one the interest paid would be:
Interest Payment Year 1 = $30,000 * (.05/1) = $1,500
This changes every pay period. So, in year 5, this would be the interest payment:
Interest Payment Year 5 = $6,599.28 * (.05/1) = $329.96
The principal payment is found by subtracting the interest payment in a given period from the fixed payment. In year 1, this would be the principal payment:
Principal Payment Year 1 = $6,929.24 - $1,500 = $5,429.24
This also changes each pay period since it is dependent on the interest payment. For the final payment in year 5, this would be the principal payment:
Principal Payment Year 5 = $6,929.24 - $329.96 = $6,599.28
The ending period balance shows how much of the principal is left after each payment is made. It is found by subtracting the principal payment amount from the beginning period balance.
In this example, the total amount paid ends up being $34,646.22, with $30,000 (the original loan amount) going towards the principal and $4,646.22 going towards interest. Due to interest on the loan, the amount paid will be more than the initial loan. The initial loan amount, the interest rate, the length of the loan, and the number of payments per year all impact how much more will be paid over that initial amount.
|
Year |
Period |
Beginning of period balance |
Fixed payment amount |
Interest paid |
Principal paid |
Ending period balance |
|
1 |
1 |
$30,000 |
$6,929.24 |
$1,500.00 |
$5,429.24 |
$24,570.76 |
|
2 |
1 |
$24,570.76 |
$6,929.24 |
$1,228.54 |
$5,700.71 |
$18,870.05 |
|
3 |
1 |
$18,870.05 |
$6,929.24 |
$943.50 |
$5,985.74 |
$12,884.31 |
|
4 |
1 |
$12,884.31 |
$6,929.24 |
$644.22 |
$6,285.03 |
$6,599.28 |
|
5 |
1 |
$6,599.28 |
$6,929.24 |
$329.96 |
$6,599.28 |
$0.00 |
|
Total Paid |
|
$34,646.22 |
$4,646.22 |
$30,000.00 |
Loan Amortization Example 2
In this example, we will look at how the length of the loan impacts the total amount paid. The producer is still looking at getting a $30,000 loan with a 5% interest rate where payments are made annually. But this time the loan will be paid out over 10 years. The loan amortization schedule in this example is shown in Table 2.
The fixed payment amount would be calculated in this way:
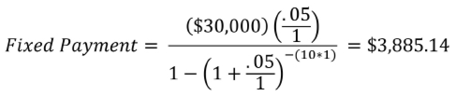
Since this loan is for 10 years paid annually, there would be 10 total payments made. Each individual payment is less than what would be paid for a 5-year loan. However, total payments would equal $38,851.37. So, while each payment is less, the producer would pay $4,205.15 more over the life of the loan with this 10-year loan compared to the 5-year loan. The reason for this is that smaller payments pay less of the principal with each payment. Therefore, interest accrues more over time. In order to not pay more with a 10-year loan compared to a 5-year loan, the producer would need to receive a lower interest rate.
|
Year |
Period |
Beginning of period balance |
Fixed payment amount |
Interest paid |
Principal paid |
Ending period balance |
|
1 |
1 |
$30,000 |
$3,885.14 |
$1,500.00 |
$2,385.14 |
$27,614.86 |
|
2 |
1 |
$27,614.86 |
$3,885.14 |
$1,380.74 |
$2,504.39 |
$25,110.47 |
|
3 |
1 |
$25,110.47 |
$3,885.14 |
$1,255.52 |
$2,629.61 |
$22,480.85 |
|
4 |
1 |
$22,480.85 |
$3,885.14 |
$1,124.04 |
$2,761.09 |
$19,719.76 |
|
5 |
1 |
$19,719.76 |
$3,885.14 |
$985.99 |
$2,899.15 |
$16,820.61 |
|
6 |
1 |
$16,820.61 |
$3,885.14 |
$841.03 |
$3,044.11 |
$13,776.50 |
|
7 |
1 |
$13,776.50 |
$3,885.14 |
$688.83 |
$3,196.31 |
$10,580.19 |
|
8 |
1 |
$10,580.19 |
$3,885.14 |
$529.01 |
$3,356.13 |
$7,224.06 |
|
9 |
1 |
$7,224.06 |
$3,885.14 |
$361.20 |
$3,523.93 |
$3,700.13 |
|
10 |
1 |
$3,700.13 |
$3,885.14 |
$185.01 |
$3,700.13 |
$0.00 |
|
Total |
|
$38,851.37 |
$8,851.37 |
$30,000.00 |
Loan Amortization Example 3
Larger purchases, such as housing, usually have a longer and more complex schedule than the above examples. This example looks at a $100,000 mortgage. As of February 2023, the average interest rate for 30-year fixed rate mortgage was 7% in Mississippi, so we used this number for the example. The amortization schedule for this mortgage is shown is Table 3.
The fixed payment is found by the same formula from before and would be calculated in this way:
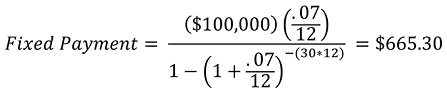
The amount paid every month would be $665.30, and this same amount would be paid for 360 months (30 years times 12 months per year). The interest and principal paid each month would be calculated using theses formulas.
In this example, the buyer would end up paying $239,508.90 to pay off the initial $100,000 loan. The interest paid over the lifetime of the loan was actually greater than that of the initial loan. This is caused by the length of the loan and the relatively high interest rate.
|
Year |
Period |
Beginning of period balance |
Fixed payment amount |
Interest paid |
Principal paid |
Ending period balance |
|
1 |
1 |
$100,000 |
$665.30 |
$583.33 |
$81.97 |
$99,918.03 |
|
1 |
2 |
$99,918.03 |
$665.30 |
$582.86 |
$82.45 |
$99,835.58 |
|
1 |
3 |
$99,835.58 |
$665.30 |
$582.37 |
$82.93 |
$99,752.66 |
|
1 |
4 |
$99,752.66 |
$665.30 |
$581.89 |
$83.41 |
$99,669.24 |
|
1 |
5 |
$99,669.24 |
$665.30 |
$581.40 |
$83.90 |
$99,585.34 |
|
30 |
357 |
$2,622.85 |
$665.30 |
$15.30 |
$650.00 |
$1,972.85 |
|
30 |
358 |
$1,972.85 |
$665.30 |
$11.51 |
$653.79 |
$1,319.05 |
|
30 |
359 |
$1,319.05 |
$665.30 |
$7.69 |
$657.61 |
$661.44 |
|
30 |
360 |
$661.44 |
$665.30 |
$3.86 |
$661.44 |
$0.00 |
|
Total |
|
$239,508.90 |
$139,508.90 |
$100,000.00 |
Reducing Overall Loan Payment Amount
The question is, then, are there ways to reduce the overall payment amount? The answer is, yes. First, you can search for a lender who will charge you a lower interest rate, of course. However, it should be noted that the rates you will receive from different lenders will be very similar to each other. But, especially for longer loans, any reduction in interest rates can significantly reduce the total amount paid.
An alternative and more effective way to reduce the payment amount is to take a loan with shorter payment schedule. This can greatly reduce the overall loan payment amount but will incur a greater monthly payment, as was shown in Examples 1 and 2. For loan amortization, the longer the payment period, the greater the amount you spend on the interest, even though the regular payment on a monthly basis might be lower.
From the lender’s perspective, the interest payment is where they make profit. For this reason, lenders do not want to see their borrowers pay off long-term loans right away. The risk of a borrower paying off payments earlier than the expected schedule is called as a prepayment risk for lending institutions, and it is why lenders will sometimes charge you a prepaid penalty if you decide to prepay loans. However, if there is no prepaid penalty then making additional payments during the life of the loan can greatly reduce the overall total paid.
One other option of reducing the overall payment amount is to put down a larger down payment. Since interest is dependent on the principal, any reduction in the initial loan amount will have a large effect on the amount of interest that accrues and subsequently the total amount paid on the loan.
Things to Consider
Running a farm business can be expensive. In fact, running any business is expensive. While borrowing money from lending institutions almost seems inevitable, it must be noted that there can be significant costs associated with that. Before taking loans, consider the following:
- Evaluate the magnitude of the regular loan payments on business cashflow.
- Evaluate whether your business will have enough cash or cash-equivalents to pay off the regular payment not just for the short term but also for the long term.
- Assess the payment level and the risk associated between floating interest rate vs. fixed interest rate at the beginning. Again, lending institutions might give you floating interest rate that will be slightly lower than the fixed rate, but there will be a risk associated with the floating interest rate as payments can change.
If you would like to evaluate a loan amortization schedule and the total costs, there are several websites where you can change the underlying assumptions. Mississippi State Extension and the Department of Agricultural Economics have developed a free loan amortization calculator Excel tool. The Farm Credit Services of America also provides a free-online page for loan amortization calculation.
Lastly, farm financial statements can greatly help in measuring the impact of loan payments on the farm’s cashflow, liquidity, and solvency. MSU Extension publications on the subject can be used to measure these changes (P3709, Managing Farm Finances in Turbulent Times; P3713, Balance Sheet; P3710, Cash Flow Statement; P3707, Income Statement; and P3712, Ratios to Measure Farm Financial Health)).
References
Barry, P. J., and Ellinger, P. N. (2012). Financial Management in Agriculture. Prentice Hall.
Farm Credit Services of America. Loan Payment Calculator.
Publication 3920 (POD-06-23)
By Kevin N. Kim, PhD, Assistant Professor, Department of Agricultural Economics; and Brian E. Mills, PhD, Assistant Professor, Agricultural Economics.
The Mississippi State University Extension Service is working to ensure all web content is accessible to all users. If you need assistance accessing any of our content, please email the webteam or call 662-325-2262.







